Measure the Speed of Light with Chocloate and a Microwave

The Speed of Light

Now anyone can measure this speed - with chocolate and a microwave oven!

The only equipment you need for this experiment is a microwave, a ruler and chocolate, cheese or any other food that melts. Remove the turntable from the microwave and replace with chocolate on a plate (so the plate does not rotate), and heat until it just starts to melt - about 20 seconds, depending on the power of the oven. There will be some melted hot spots and some cold solid spots in the chocolate. The distance between the hot spots is half the wavelength of the microwaves, and the frequency of the microwaves will often be printed on the back of the oven. The speed of light is equal to the wavelength multiplied by the frequency of an electromagnetic wave (microwaves and visible light are both examples of electromagnetic waves). So from this simple experiment, and some easy math, you can work out the speed of light from Milky Way Magic Stars®!

How it works:

When you turn on your microwave oven, electrical circuits inside start generating microwaves – electromagnetic waves with frequencies (which we'll call "f" later) around 2.5 gigahertz – 2500000000 Hz. These waves bounce back and forth between the walls of the oven, the size of which is chosen so that the peaks and troughs of the reflected waves line up with the incoming waves and form a “standing wave”.

If you pluck a guitar string, you’ll set it vibrating. Usually, you will excite the “first harmo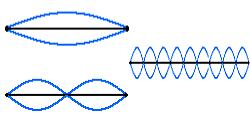nic” – a standing wave that has the string stationary at the bridge and the fret, and vibrating back and forth in the center. With effort, you might be able to excite the second harmonic (try plucking the string in opposite directions ¼ of the way in from either end), then you’ll see the string vibrating back and forth, with the center stationary. This pattern has three nodes or points with no displacement away from rest (there’s a useful mnemonic - a NODe has NO Displacement): the ends and the center, and two anti-nodes: ¼ and ¾ of the way along its length. There are infinitely many modes, one for each positive integer, with more and more nodes between the fret and the bridge.

A full wave is shaped like a “sine function” going from zero to a maximum back through zero to a negative maximum and back to zero again - like the second harmonic in the figure on this page. So you can see that the distance between the maximum displacements of the wave is one half the wavelength.

The electromagnetic field inside the microwave behaves in roughly the same way – except the vibrations are in “the electromagnetic field”. Where the vibrations are greatest (the anti nodes), you will see the greatest heating, but at the nodes, the chocolate will only melt slowly as heat diffuses into those areas.

Thus, the distance between the melted regions (x) is equal to the distance between the antinodes, and equal to half the wavelength (λ)!

So, the detailed calculation to find the speed of light (c) is:

c=λ*f

c=2*x*f

Ever eager to confirm things for himself, your guide conducted this experiment in his kitchen. In order to protect my microwave, I took the probably unnecessary (but I would recommend doing this to everyone) precaution of placing a half glass of water in the microwave – if there is insufficient material in a microwave, you can blow the internal fuses, rendering the microwave inoperable. However, as the microwave then had to heat the water as well, the melting process took almost two minutes, rather than twenty seconds.

Once I took the chocolate out of the microwave (not having access to Milky Way Stars, I used a block of Ghirardelli semisweet chocolate – a solid block of chocolate conducts heat along its length more than a collection of small chocolates, so I would recommend using some sort of chocolate chips when you do this your self) I measured the distance between the melted points from my sample was 6cm.

As my microwave didn’t have a frequency reading on the back, I will use the 2.5GHz “typical” value I found after a brief web search.

Thus: the wavelength is .06m x 2 = 0.12m Then the speed is 0.12m x 2.5 x 10⁹ /s = 3 x 10⁸ m/s, which is a pretty good estimate! If you want to do better, you can try repeating the measurement many times (and making very accurate measurements) and applying statistics to get an average, and an estimate of how much uncertainty you have.

Pablo Coronel, of the Food Science Department at North Carolina State University, has sent me a few more suggestions like this one:

1. Marshmallows, they swell when heated in the Mico-Wave oven, so it can be fun.

2. Fax paper, the thermal type, place a piece of cardboard and a wet (but not soaked) paper towel beneath the fax paper. There will be dark spots where the antinodes are.

Another reader also recalled having, as a microwave technician, tested the wavelength of microwaves using a small bulb and a short piece of wire connecting the bulb's terminals. If the wire is at a node, then there will be no current in the wire and the bulb will not glow. However, if the bulb is away from the nodes and is aligned parallel to the direction of the electromagnetic field at that point, the field will cause a current to flow in the wire, lighting the bulb. The bulb will light brightest at the anti-node. However, I do not recommend trying this at home, as metal objects should not be placed in the microwave.

Nothing travels faster than light - it only takes 8 minutes for it to reach the Earth from the nearest star, the Sun, which is 150 million kilometers away. This means that when you see the sun (remember not look directly at the sun), you’re really seeing light that left the sun 8 minutes ago – you’re seeing the sun as it was, and where it was, 8 minutes earlier.

One of the first to try to measure the speed of light was Galileo: In the early 17th century, the general belief amongst scientists (or natural philosophers as they were often called then) was that the speed of light was infinite; that is, light could travel any distance in no time at all. Just as with many other important discoveries made by Galileo, he disagreed with most of his contemporaries. One of Galileo’s great strengths as a scientist was his ability to conceive experiments to test his theories. To measure the speed of light, he and his assistant each took a shuttered lantern to hilltops one mile apart. Galileo flashed his lantern, and the assistant was supposed to open the shutter to his own lantern as soon as he saw Galileo's light. Galileo would then time how long it took before he saw the light from the other hilltop. Then, he could divide the distance by the time he measured to get a speed.

Unfortunately for Galileo, this time he had not conceived an experiment sufficiently clever to measure the extraordinary speed with which light traveled. We now know that light travels at approximately 3x10⁸ m/s (that is approximately 1100000000 km/Hr), so it would travel the one mile (1.6 km) between the hills in 0.000005 s (5 microseconds), whereas, even if Galileo could time such a short trip, the assistant could not possibly unshutter his lantern fast enough that Galileo could tell what part of his measurement was the travel time!

In fact, due to the extraordinarily high value of c (c is the standard symbol physicists use for the speed of light), there was no where on earth any two people could stand so that they could conduct this experiment. In order to make such a direct measurement of the speed of light, one needed a laboratory much larger than the earth! Remarkably, Galileo had effectively created such a Laboratory with another of his discoveries – the moons of Jupiter.

In the 1676, Danish Astronomer Ole Roemer made the first reasonable observation of the finite speed of light. Since Galileo’s discovery of the larger Jovian moons, a great deal of telescopic observation lead to extremely precise measurements of the orbital period of Io (1.76 Days). Testing these calculations, Roemer observed the eclipses over the course of a Jovian year. Roemer found that as Jupiter moved further from the Earth, his predictions of when Jupiter’s moons would cross its face became less and less accurate. The times that he saw Io cross the face of Jupiter became steadily later than the times predicted, as much as one and a quarter hours late. However, as he continued to observe, he was able to discount the idea that his predictions were simply wrong, as on its approach to the earth, the events once again approached his predicted times. In a stroke of genius, Roemer attributed this discrepancy to the finite speed of light, and he even published an estimate of that speed, approximately two thirds the currently accepted value (due to the inaccurate estimates of the size of Earth and Jupiter’s orbits of his contemporaries). With the correct measurements of the orbits, Roemer’s data gives a speed of light equal to 3x10⁸ m/s.

In an unwitting homage to Roemer, the motion of Jupiter now seems to have allowed measurement of the speed gravity propagates.

Since then, the speed of light has been measured in many ways – as technology increased, the speed became easier and easier to tap into. In the 1920’s Fizeau and Foucault (of Pendulum fame) in France competed to measure the speed of light using high speed rotating objects. Foucault bounced light from a stationary mirror to a mirror that was quickly rotating – the angle the light was reflected through allowed a precise measurement of the speed of light. Eventually, Foucault was able to determine that light traveled at 299,796 Km/s, an extraordinarily accurate value.

Even more recently, we have been able to essentially conduct Galileo’s Experiment in two ways – Using high speed electronics and long lengths of optical fiber in a laboratory, or by timing Laser light reflected off mirrors placed on the moon by the Apollo astronauts.

Early in the 20th century, Einstein’s special theory of relativity established the speed of light not just as finite value, but also a universal constant and “speed limit”.